I thought my earlier essay on .999… did a pretty good of explaining why I (along with 99.999…% of mathematicians) say that it equals 1, until I asked some of my students what they got out of it; then I got a humbling jolt of pedagogical reality. The students agreed that .999… is the limit of the sequence .9, .99, .999, etc., and they also agreed that the limit of that sequence is 1. So you might think that they would have agreed that .999… equals 1, but no: they couldn’t swallow that conclusion.
I’ve decided that part of what’s going on is that my students arrive at college with a number-sense that’s so deeply grounded in their experience with terminating and non-terminating decimals, in worksheet after worksheet, that these concrete representations have taken on an independent reality for them. At that point, it does little good to tell them “.999… doesn’t mean anything till we assign it a meaning” or “We’re going to define .999… as a limit”, because they already “know” what .999… is: a dot followed by infinitely many 9s! No attempt to redefine .999… can shake loose their sense of what it already means to them.
So today I’m going to come at the problem of .999… from a totally different direction. The fork in the road is a statement from my first essay: “I thought there was a need for an explanation that confronts the question of what a non-terminating decimal like .999… means, instead of just juggling it using plausible rules grounded in the behavior of terminating decimals.” Let’s flip that. Instead of exploring what .999… could or should mean, let’s embrace those plausible rules for operating on infinite decimals and see what they lead to!
I don’t think I’ll change anyone’s mind today, but I hope to point readers’ eyes toward a neutral ground that accommodates both ways of interpreting .999…
THE PLAYING FIELD
Before we can start playing with infinite decimals, we need to agree on some basics. Sometimes one uses “…” to refer to a finite stretch (as in “1,2,…,100”), but that’s not the game we’re playing here: by “.999…” I mean a decimal point with infinitely many 9s after it. Since the “…” never ends, we can’t talk about the “last” 9. In particular, there’s no “infinity-eth” 9; there’s an nth 9 for every counting number n, but infinity isn’t a counting number.
We also need to rule out things like .999…7. You could use the theory of transfinite ordinals to cook up a number system that would allow you to imagine a 7 that appears after an infinite sequence of 9’s, but we’re not allowing such things in the infinite decimals game. Remember where infinite decimals come from: the task of trying to divide 1 by 3 (say) and failing to finish in finite time. In that context, “…” means “and so on, forever”, so it makes no sense to write something after the “…”.
GETTING STARTED
So now let’s play! Say we want to add .666… to itself. What do we do? You may have learned that you’re supposed to add digits from right to left, starting with the rightmost digit, but how can we do that when there is no rightmost digit?
The answer is simple: add left to right! Before we add infinite decimals left-to-right, let’s warm up by adding ordinary whole numbers left-to-right. For instance, suppose that we’re adding 25 (that’s 20 plus 5) and 36 (that’s 30 plus 6). Going from left to right, we add the 20 and the 30 to get 50, and we add the 5 and the 6 to get 11, so our answer is fifty-eleven, better known as sixty-one. Let’s put that calculation in the grid-format of the standard algorithm:
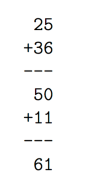 If you prefer we can leave out the 0 after the 5 (since the positioning of the 5 in the tens place already tells us that it stands for 5 tens):
If you prefer we can leave out the 0 after the 5 (since the positioning of the 5 in the tens place already tells us that it stands for 5 tens):
 (I’ve also left out the second plus sign this time, trusting you to remember that we have to add.)
(I’ve also left out the second plus sign this time, trusting you to remember that we have to add.)
Here’s another example of adding finite numerals with digits going from left to right, this time using decimals:
 Likewise, .6666 plus .6666 is 1.3332, and so on.
Likewise, .6666 plus .6666 is 1.3332, and so on.
You’ll notice my use of the infamous word numeral. For a while, the distinction between numbers and numerals was thought by some people to epitomize the sterile pedantry of 1960s-era New Math. But I think that it’s a valuable distinction that should be taught as early as possible, and I’ll say why later.
GOING INFINITE
Now let’s use this left-to-right approach to add 2/3 + 2/3 in decimal:
 The lone 2 that we saw at the end of .666+.666 and at the end of .6666+.6666 is gone; it’s disappeared off to the right. Is it still somehow there, “at infinity”? Is .666… plus .666… equal to 1.333…2? That can’t be; we’ve already agreed that you can’t write digits after the “…”. It seems strange that the 2 is gone, but that’s the way this game this played. Also, we want 2/3 plus 2/3 to equal 4/3, otherwise known as 1 plus 1/3, whose decimal expansion is 1.333…; it would be a poor theory of infinite decimals that can’t reproduce basic facts about fractions.
The lone 2 that we saw at the end of .666+.666 and at the end of .6666+.6666 is gone; it’s disappeared off to the right. Is it still somehow there, “at infinity”? Is .666… plus .666… equal to 1.333…2? That can’t be; we’ve already agreed that you can’t write digits after the “…”. It seems strange that the 2 is gone, but that’s the way this game this played. Also, we want 2/3 plus 2/3 to equal 4/3, otherwise known as 1 plus 1/3, whose decimal expansion is 1.333…; it would be a poor theory of infinite decimals that can’t reproduce basic facts about fractions.
Now that we’re comfortable with adding infinite decimals from left to right, we can add .999… to itself:
 So when you double .999…, you get 1.999… (not 1.999…8 or anything else).
So when you double .999…, you get 1.999… (not 1.999…8 or anything else).
If you want to really challenge yourself, trying using left-to-right operations to multiply .999… by 9, or to multiply .999… by itself; see Endnote #3 for answers.
EXPLODING DOTS
A helpful device for thinking about these operations is a kind of infinite abacus recently popularized by mathematician-at-large James Tanton. (“Mathematician-at-large” is actually his official job title; he works for the Mathematical Association of America, and his job is spreading the joy of mathematics to learners of all ages, especially young ones.) Tanton calls the device Exploding Dots. Here’s how Tanton would represent the infinite decimal 0.999…:
 Here’s how he would represent what you get when you add 0.999… to itself:
Here’s how he would represent what you get when you add 0.999… to itself:
 (Note that the number of dots in each box has doubled, from nine to eighteen.) But that’s just the first position in our Exploding Dots game. Any time you see a box with ten or more dots in it, you’re free to take ten dots from that box and replace them by a single dot in the box immediately to the left, like so:
(Note that the number of dots in each box has doubled, from nine to eighteen.) But that’s just the first position in our Exploding Dots game. Any time you see a box with ten or more dots in it, you’re free to take ten dots from that box and replace them by a single dot in the box immediately to the left, like so:
 In Tanton’s metaphor, having ten or more dots in a box causes an unstable situation that, sooner or later, has to result in an explosion that sends a dot to the left (and removes nine dots from the board).
In Tanton’s metaphor, having ten or more dots in a box causes an unstable situation that, sooner or later, has to result in an explosion that sends a dot to the left (and removes nine dots from the board).
Oh, but the new position I just showed you still has lots of instabilities (infinitely many, in fact). Rather than explode the instabilities sequentially (which would take forever), let’s explode them all simultaneously:
 No more explosions are possible, so our process of simplification is done. This is the Exploding Dots way of showing that .999… plus .999… equals 1.999…
No more explosions are possible, so our process of simplification is done. This is the Exploding Dots way of showing that .999… plus .999… equals 1.999…
In the Exploding Dots game, you’re allowed both explosions and unexplosions, where an unexplosion takes a single dot in a box and replaces it by ten dots in the box to its right. You’re even allowed to carry out infinitely many of these operations. Exploding Dots is a beautiful self-consistent mathematical system, and Tanton has created a whole bunch of on-line lessons that use them. Millions of people are going to be learning about Exploding Dots as part of Global Math Week this coming October.
For all its strengths, Exploding Dots (or rather the version that I’ve described so far) has an important limitation: there’s no way to start from 0.999… and, by performing explosions and unexplosions, turn it into 1.000… In fact, there is a self-consistent, nonstandard version of the infinite decimal system in which 0.999… and 1.000… are different entities. In such a nonstandard system, you might call 0.999… the evil twin of 1.000…; the fault-line in the number system that separates them may be infinitesimal, but it still separates them. Ditto for 1.999… and 2.000… Many other numbers have evil twins: for instance, 1/2, aka .500…, has .499… as its evil twin, and 1/5, aka .200…, has .199… as its evil twin. But 1/3 aka .333… has no evil twin. (Remember, .333…2 and 333…4 and the like aren’t permitted in this game.)
I don’t know if this kind of arithmetic has a name, so I’ll dub it the literal decimal system. The fact that the literal decimal system is self-consistent and has intuitive appeal might lead you to ask why we don’t teach it to kids alongside the standard theory of infinite decimals.
VISITING MARS
To answer that question, let’s visit the Mars of H.G. Wells’ War of the Worlds, which is inhabited by aliens with three fingers on each of their two hands. I’ll assume that the Martians have given up on the project of invading Earth, and that the two species have gotten so chummy that they’re sharing arcane aspects of their respective planetary cultures. We’ve learned that Martians use place value based on powers of the number six, and that they accordingly use six digits, which we’ll write as 0,1,2,3,4,5 even though Martian use different symbols. For instance, the number nine would be written in Martian as 13, meaning 1 six plus 3 ones, and the fraction one-half would be written as .3, meaning 3 sixths.
The Martians have told us that, like us, they have families and cars, and that young Martian kids get very excited when the odometer in the family car ends in a whole bunch of 5s, because the kids have learned that those 5’s are about to roll over into a bunch of 0s: the numbers 555555 and 1000000 are dramatically different, even though they differ by only 1. Consequently, when they get a little older and attend Martian middle school and learn about infinite heximals, Martian kids often balk at .555… being equal to 1, even though their teachers insist that it’s true.
A Martian mathematician has written an essay about this, very similar to the one you’re reading now, but that other essay uses base six instead of base ten. In talking about Exploding Dots, my Martian counterpart discusses a device in which you can trade six dots in any box for a single dot one box to the left, and vice versa. Also, her discussion of evil twins is different from mine. On Earth, as we saw in the last section, that the fraction 1/5 has an evil twin but the fraction 1/3 doesn’t. On Mars, 1/3 has an evil twin but 1/5 doesn’t! (See Endnote #6.)
It bothers both me and my Martian colleague that the number 1/3 has an evil twin on Mars (but not on Earth) whereas the number 1/5 has an evil twin on Earth (but not on Mars). We like to think that underneath all the ways we write numbers, there’s something universal, not planet-specific. Earthlings may count 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, … where Martians counts 1, 2, 3, 4, 5, 10, 11, 12, 13, 14, 15, 20, 21, …, but both species are talking about the same thing. We can create a one-to-one correspondence between our numbers and the Martian counting numbers. But when it comes to infinite decimals and infinite heximals, evil twins ruin the correspondence between the two systems.
And now you can understand one component of my quarrel with the literal decimal system: it’s so darn parochial! When you enthrone the decimal system as the embodiment of the concept of real numbers, you’re committing yourself to a number system whose properties depend on how many fingers you have. And I don’t like that, because I believe that whether or not there are three-fingered Martians, the laws of physics are going to be the same on Mars as they are on Earth, so the number system that we use to write the laws of physics shouldn’t depend on how we represent those numbers.
I have other problems with the literal decimal system; see Endnote #7. All these difficulties can be fixed if we get out our mathematical “glue” gun. Gluing is a way to create new number systems from old. For instance, we can create mod 2 arithmetic (related to but different from base 2 arithmetic) if we glue all the even integers together (and call the result Even) and we glue all the odd integers together (and call the result Odd), obtaining a new number system that has only two elements in it called Even and Odd, satisfying relations like “Even plus Odd equals Odd” and “Even times Odd equals Even”. We can do something similar to the literal decimal system: glue each number to its evil twin. If the Martians get out their glue-guns and apply the same kind of gluing with their evil twins, they get a glued-together number system that is the same as our glued-together number system! For instance, if they agree that and
are “really” the same thing, then we no longer have the problematic situation that they have two numerals (.200… and .155…) that correspond to just one of ours (.333…). Likewise, if we agree that
and
are “really” the same thing, then we no longer have the situation that the number 1/5 corresponds to two Earth-numerals but only one Mars-numeral.
Martian heximals and our decimals now emerge into the light as two different systems for representing the same underlying structure, which mathematicians call the field of real numbers.
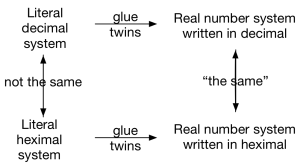 Here I’ve put “the same” in quotes, to emphasize that I’m using the term in a specialized sense, meaning that we can put them into one-to-one correspondence. (Actually, I mean something more than this: I mean that the two number-systems are isomorphic. But isomorphism is a whole topic unto itself.)
Here I’ve put “the same” in quotes, to emphasize that I’m using the term in a specialized sense, meaning that we can put them into one-to-one correspondence. (Actually, I mean something more than this: I mean that the two number-systems are isomorphic. But isomorphism is a whole topic unto itself.)
My view of the literal decimal system can be summarized as my feeling that it is not as beautiful as the real number system. The standard explanation for why we don’t teach this system would be that this number system is not as useful. But the two explanations are linked: in mathematics the useful and the beautiful coincide more often than we know how to explain.
There are articles that use the literal decimal system as a springboard for constructing the real numbers; see the article by Richman, fittingly published at the tail end of 1999, and the older article by Faltin, Metropolis, Ross, and Rota, both listed in the References.
A more recent article by Katz and Katz lingers on the question of “unital evaluation of .999…” (that is, the question of whether .999… = 1); without building up the literal decimal system explicitly the way Richman does, their article explores contexts in which it’s correct to view .999… as being different from 1.000… Of course these contexts are not the standard real number system.
WHAT TO TELL THE KIDS
When we answer kids’ questions about the facts of infinite decimals, as when answering their questions about the facts of human reproduction, it’s not a good idea to explain everything we know. So what do we say? Here I’d welcome comments from people who actually teach math at the precollege level and have experience with this issue. Is a teacher ethically required to answer every question that a student asks? Or is evasion sometimes the best reply?
One thing I’ll suggest is that it might be a bad idea to introduce the topic to students who aren’t worried about it to begin with. Some students will think “Why does it matter whether or not .999… equals 1?” (one of the students I polled about the issue was bold enough to ask this question in class), and I suggest that those students shouldn’t be required to participate in such discussions until they have developed their own disquiet about the issue. It’s one thing to have someone scratch you where you itch, and it can be quite a nice thing indeed, but for someone to scratch an itch that you don’t have can be annoying.
Here’s what Katz and Katz have to say about the issue of unital evaluation of .999…:
We have argued that the students are being needlessly confused by a premature emphasis on the unital evaluation, and that their persistent intuition that .999… can fall short of 1, can be rigorously justified. Other interpretations (than the unital evaluation) of the symbol .999… are possible, that are more in line with the students’ naive initial intuition, persistently reported by teachers. From this viewpoint, attempts to inculcate the equality .999… = 1 in a teaching environment prior to the introduction of limits appear to be premature.
I would add, following up on Ed Dubinsky’s ideas about mathematical learning, that more mathematically sophisticated learners have two conceptions of .999… (one as an unending process, and one as an encapsulation of that process) whereas more mathematically naive learners have only one (as an unending process) and that the latter also also metacognitively fail to appreciate that the former have something in their heads that they themselves lack.
Here I’m reminded of the way young kids think about conservation of matter. The psychologist Piaget famously theorized that there’s a natural developmental progression from thinking that a taller container must hold more liquid than a shorter container (even when the shorter container is wider) to a more nuanced understanding of volume, mediated by the notion of conservation: if you can pour all the liquid from one container into another, they must contain the same amount of liquid. Artificial intelligence pioneer Marvin Minsky wondered whether this developmental progression could be accelerated by appropriate tutelage, and tried to coach his kids in the ways fluids behave. He sort of succeeded, and sort of didn’t. When Minsky’s friend the psychologist Gilbert Voyat (then a student of Piaget) met Minsky’s five-year-old kids Julie and Henry, he thought it was a perfect opportunity to test out Piaget’s ideas. He performed Piaget’s water jar experiment, and asked Julie “Is there more water in this jar or in that jar?” Julie answered (incorrectly) “It looks like there’s more in that one,” then adding, “But you should ask my brother, Henry; he has conservation already.”
Julie may have lacked the notion of conservation, but (thanks to her father) she had something even more astonishing in a five-year-old: a precocious metacognitive appreciation of her future developmental stages. I suppose we could hope for something analogous in math pedagogy: young Julies who’ll say “Well, I think .999… is different from 1, but you should really ask Henry, because he already has encapsulation.” But that’s an awful lot to expect from a kid!
Maybe the best approach is to evade the question and encourage productive controversy, with good-humored reticence. As a parent I do this kind of thing all the time. When my son was fishing for answers to questions about the Harry Potter books (“Snape turns out to be evil, right?” and “Harry and Hermione get married at the end, don’t they?”), I brought my best poker-face to the conversation, and got all Rogerian on the poor kid: “Interesting theory. Why do you believe that?” It’s a tricky road to tread, especially when you switch from the magical world of J. K. Rowling to the mundane world of math pedagogy: we don’t want to mislead kids into thinking that anything goes when it comes to .999… versus 1, but we don’t want to tell them the answer either. At least, I don’t! I think an unanswered question can be a wonderful thing, and a person unable to tolerate suspense is the poorer for this intolerance.
I also think that a good way to deal with the .999… issue is to cunningly lay the groundwork for it by teaching kids the difference between numbers and numerals at an early age. When we teach about Roman numerals, we should say “The Roman numeral XVII represents the same number as the Arabic numeral 17”. This distinction lays the groundwork for a more philosophically mature way of thinking about .999… and 1.000: instead of asking “Are they the same?”, students can ask the more nuanced question “Do these two numerals correspond to the same real number?” This will make it easier for kids to accept that there’s something problematical about .999… in the first place: since it’s just a numeral, there’s a chance that, like the Roman numeral IVX, it might turn out not to mean anything at all. Alternatively, just as IIII and IV mean the same thing in Roman numerals, it becomes thinkable that two decimal numerals that look different might turn out to be equal.
I like to imagine that a kid who starts out thinking that .999… and 1 are different numbers, and then finds out they’re the same, could have the same frisson that I had back in 1980 when, along with millions of other moviegoers, I found out that Luke Skywalker’s father and Darth Vader were the same person. Yeah, George Lucas had fooled me, but the payoff was worth it. So maybe it wouldn’t be so bad to leave kids in suspense about whether .999… and 1 are the same number?
Next time: Minus infinity.
Thanks to Joerg Arndt, David Jacobi, Evelyn Lamb, Henry Picciotto, Fred Richman, and James Tanton.
ENDNOTES:
#1: I had my students read my earlier essay and then I took a vote — I put several propositions on the board and asked them to classify them as “Agree” / “Disagree” / “Don’t understand”. Here’s how it broke down:
0.999… equals 1:
8 agreed, 16 disagreed, 4 didn’t understand
0.999… gets closer and closer to 1, but never equals it:
16 agreed, 1 disagreed, 8 didn’t understand
The numbers 0.9, 0.99, 0.999, 0.999, … get closer and closer to 1:
21 agreed, 0 disagreed, 1 didn’t understand
The limit of the sequence (0.9,0.99,0.999,…) equals 1:
24 agreed, 0 disagreed, 0 didn’t understand
0.999… equals the limit of the sequence (0.9,0.99,0.999,…):
6 agreed, 17 disagreed, 6 didn’t understand
Someone who knows more about pedagogy than I do should ponder how this poll gives us insight into students’ thought processes.
#2: Joerg Arndt, in response to my earlier essay, wrote:
I found the following a good/easy way to convince people that 0.99999… = 1:
0.11111… = 1/9
0.22222… = 2/9
0.33333… = 3/9
0.44444… = 4/9
…
0.99999… = 9/9 = 1
It’s funny how some people then say “Oh, that’s right!”
I think the reason Joerg found this funny is that those same students weren’t convinced by the shorter argument that says “Multiplying both sides of the equation 1/9 = .111… by 9 gives the equation 9/9 = .999…”, even though it’s basically the same argument. It’s only when he supports this argument by citing the (arguably irrelevant) equations 2/9 = .222…, 3/9 = .333…, etc. that students find this argument convincing.
This is an application of the “majority rule” principle of math-by-pattern-matching, and I can see how that would convince people (even though I hate to resort to tricks like this myself). There’s some cynical wisdom here about how to win arguments: Artificially increase the amount of evidence you bring to support your position, so that it overwhelms counterarguments by sheer quantity. People may say “Well, there are nine arguments in favor of Proposition X and only one argument against it, so I guess I should accept it,” even if the nine arguments are basically the same argument in different guise. It’s the mathematical equivalent of the shady trial law trick of wordlessly slamming down a thick stack of papers on the table, hoping the jury will mistake it for a mountain of evidence that supports your position.
#3: Here’s how we can multiply .999… by 9:
 And here’s how we can use that to multiply .999… by itself:
And here’s how we can use that to multiply .999… by itself:
 So .999… times .999… equals .999….
So .999… times .999… equals .999….
Actually, I’m glossing over some details here, as you’ll see if you carry out the calculation a bit farther to the right. If you go far enough out, you’ll see digits that don’t add up to 9; for instance, there’s a 0 and an 8 that add up to only 8, but then to the right of that, there’s a 9, a 9 and a 1 that add up to 19:
 So if we really want to do this properly, we need another round of grouping. And if you go even farther out, you’ll find another place where you get an 8 where you expected a 9. So saying what we mean by the product of two infinite decimals can be tricky!
So if we really want to do this properly, we need another round of grouping. And if you go even farther out, you’ll find another place where you get an 8 where you expected a 9. So saying what we mean by the product of two infinite decimals can be tricky!
#4: Exploding Dots was partly based on “chip-trading” activities that teachers have used since the 1970s to teach place value. It was also partly inspired by a talk I gave a couple of decades ago, which was in turn based on Arthur Engel’s work on his probabilistic abacus, which I mentioned in my essay on Pólya’s urn model. It’s not at all obvious how to get from probability theory to systems for representing numbers, but it’s a great story, and one I plan to tell soon.
#5: In the full version of Exploding Dots, there are also “antidots”. An antidot and a dot can come together and annihilate one another, cancelling each other out (and, going the other way, a pair consisting of a dot and an antidot can be created out of nothing, like virtual particle-pairs in a quantum vacuum). Alternatively, antidots can ignore dots and engage in exploding and anti-exploding on their own (that is, you can trade an antidot in some position for ten antidots in the next position over to the right, or vice versa). With the aid of antidots, you can actually turn 0.999… into 1.000… and vice versa: just create a single dot/antidot pair in each position to the right of the decimal point, let all the dots explode (so that ten dots in one position become a single dot in the position to the left), and lastly let dots and antidots cancel wherever possible. You’ll see that there’s just a single dot left at the end, representing 1.000…
Exploding-Dots-and-Antidots and Exploding-Dots-without-Antidots, conceived of purely as games, can be compared and contrasted in a number of ways — Which is more fun? Which is easier to think about? — but it doesn’t make sense to ask which one is “right”. They’re just games. In a parallel way, modern mathematics permits us to have formal systems in which .999… < 1.000… (the literal decimal system) and formal systems in which .999… = 1.000… (the real number system). There’s no contradiction, because the meaning of the symbols depends on what rules we’re assuming. We can compare two formal theories in a variety of ways — Which is more beautiful? Which is more interesting? Which is more useful? — but it doesn’t make sense to ask which one is “true”. It depends on the interpretation!
In the literal decimal system, we have a nice simple way of deciding which of two decimals is larger: just look at the digits from left to right. But we have to give something up: subtraction isn’t well-defined (see Endnote #7). In the real number system, subtraction is well-defined, but we lose the simplicity of left-to-right comparison. Math is full of trade-offs like this. (Another trade-off is the transition from real to complex numbers: more equations have solutions when you graduate to the complex number system, but you can’t extend the is-less-than relation in a satisfying way.) The further you go in math, the more cumbersome the literal decimal system becomes; by the time you get to calculus, it becomes unworkable.
#6: It’s easy to see that 1/3 is .2 (or .200…) in the heximal system; it’s 2 sixths. It’s harder to see why 1/5 is .111… as an infinite heximal. You could get the answer by doing division in heximal, or you could just compute the sum using the formula
with r = 1/6.
Mike Lawler made a video of his son exploring binary, and in particular, the binary expansion of 1/3.
#7: One defect of the literal decimal system is that subtraction and division become problematical. Remember the equation .999…+.999…=1.999… that we calculated earlier? Comparing that with the equation .999…+1.000…=1.999…, we see that the literal decimal system doesn’t satisfy the cancellation property for addition: that is, in the literal system you can have three numerals a, b, c (namely a=.999…, b=0.999…, and c=1.000…) where a+c equals b+c even though a is not equal to b. Without the cancellation property for addition, it’s unclear how to define subtraction. Likewise, .999…×.999… and .999…×1.000.. are both equal to .999… (see Endnote #3) so multiplicative cancellation doesn’t work either in the literal decimal system. And without multiplicative cancellation, it’s unclear how to define division.
A more fundamental problem I have with the literal decimal system is that some numbers have evil twins and others don’t. This seems deeply unfair, and also, it doesn’t correspond to my geometrical intuitions about real numbers. Real numbers correspond to points on a number-line, and every point on the line is the same as every other, so why should some points have evil twins while others don’t?
Of course we could introduce a number system in which every real number has an evil twin; that would be fair. Or while we’re at it, why not give every real number infinitely many lookalikes? That sounds crazy, but it’s essentially what’s going on in an alternative theory of called nonstandard analysis.
#8: With the perspective that the literal decimals and the real numbers are two different systems, we can think more critically about some of the arguments that prove that .999… = 1. For instance, consider the following argument: “If S = .999…, then 10S = 9.999…; subtracting the first equation from the second, we get 9S = 9, so S = 1.” This is a valid proof in the real number system, but not in the literal decimal system, because subtraction is not well-defined there (see Endnote #7).
#9: As an alternative to gluing twins together, we could banish one of them. For instance, to get from the literal decimal system to the real number system, we could forbid infinite decimals that end in an infinite string of 9s. But that’s not the only option. I once wrote a talk called “.999… is greater than 1.000…”, in which I argued (only partly in jest) that, if we have to banish one of the twins, 1.000… is the one to banish. In part, this was motivated by the theory of Abelian sandpiles, because in sandpile terminology, one might call .999… the “recurrent” version of 1.000…, and sandpile theory is especially nice when we restrict to recurrent sandpile configurations. (For more on sandpiles, check out the Numberphile video on the topic.) But here’s a down-to-earth way of arguing for the proposition that .999…is “better” than 1.000…: if you make a two-by-two addition table for what you get when you add .999… or 1.000… to .999… or 1.000…, you’ll see that three of the four entries in the table end in an infinite string of 9s.
 So, by the applying that “majority rule” principle again, we can convince ourselves that infinitely many 9s is the way to go.
So, by the applying that “majority rule” principle again, we can convince ourselves that infinitely many 9s is the way to go.
#10: What would “IVX” mean in Roman numeral? It could mean IV subtracted from X, which equals 6. But it could also mean I subtracted from VX, where VX means V subtracted from X. Then VX means 5, so IVX means 4. That is, IVX could mean either (X−V)−I or X−(V−I), which mean two different things.
#11: I think an even better reason for teaching kids about numbers and numerals is the prevention of mistaken notions of what equality means. Kids who accept 2+3 = 5 but balk at 5 = 2+3 (as some do) are thinking about equality too procedurally; they think that the meaning of “A equals B” is that by following some rules you can turn A into B. So no wonder those kids have trouble with 0.999… = 1! We want kids to understand that “2+3 = 5” means that the expressions “2+3” and “5” are two different names for the same number. That can be a hard thing to grasp, so we want to give kids a chance to learn it at many stages along the K-12 curriculum, and it helps to have the number/numeral distinction to support it. You don’t have to be a devout adherent of the Sapir-Whorf hypothesis to believe that it’s easier to understand a distinction when your language contains words for the two things being distinguished.
REFERENCES:
F. Faltin, N. Metropolis, B. Ross, and G.-C. Rota, “The real numbers as a wreath product,” Advances in Math. 16 (1975), 278-304. The title is a bit misleading; the central point of the article is that you can construct the real numbers from digital expansions, whereas the fact that one stage in the construction uses something called a wreath product is a mere technicality. Here’s a link that might work.
Karin Usadi Katz and Mikhail G. Katz, “When is .999… less than 1?”. Available at http://arxiv.org/abs/1007.3018.
Fred Richman, “Is 0.999 … = 1?”, Mathematics Magazine, Vol. 72, No. 5 (Dec., 1999), 396-400. Available at http://math.fau.edu/richman/Docs/999.pdf.

I already see one point I overlooked: without the antidots, Exploding Dots has no way of representing negative numbers. Correspondingly, the theory of “literal” infinite decimals, as I’ve presented it, is limited to positive infinite decimals. Problems ensue if one tries to bring -.999… and -1 into the game.
LikeLike
I think it’s misleading to say ” Exploding Dots is a beautiful self-consistent mathematical system”; what does “a beautiful self-consistent mathematical system” mean? The system consisting of the integers, with an operation that is just like normal addition except that 7 + 12 = 37, is a self-consistent mathematical system; it just doesn’t have the properties you expect addition to have, like commutativity and associativity. That’s what makes me say it is “less beautiful” than the normal system of addition. Similarly, If Exploding Dots lacks cancellation and inverses, that makes it less beautiful. I think it’s more honest to say specifically what properties the system does and doesn’t have, rather than just saying “it’s self-consistent and beautiful”.
Any system in which .9999…. is not equal to 1 must lack one of the following properties, and I think that any system which doesn’t have those properties is considerably less beautiful:
Property 1: .99999… * 10 = 9.999999…
Property 2: 9.99999… – .9999999 = 9.
Property 3: The distributive property, that lets us conclude that 10 * .9999… – 1* .9999… = 9 * .99999…
Which of these properties does exploding dots lack? Is subtraction even defined?
LikeLike
I could’ve been more up-front about how the bare-bones version of Exploding Dots lacks subtraction (a point that is relegated to the Endnotes). And I agree that this makes it less beautiful.
LikeLike
Your essay is colored by your affection for real numbers, and I worry that if I can “hear” your affection for the reals, then any student will also sense it, and consequently either engage in motivated search for some kind of flaw in it (which they, of course, could find), or worse, disengage from you as a teacher.
If the conclusion of the essay was not “the standard reals are here to stay”, but was instead “therefore Abraham Robinson’s infinitesimals are the right way to do things”, or Conway’s surreal numbers, or some other substantive and principled alternative to the various disgusting constructions of the real numbers, then I think the essay as a whole would be more palatable. Even if you personally can’t endorse that position, the essay could endorse it.
Is there anything more tedious and contrived than Dedekind cuts? All that work, in order to get such a mundane outcome, that simply says “shut up” to essentially every philosophical question about numbers that students have, like “what is infinity minus infinity?” and bites the bullet on all kinds of viciously nasty side-effects that a student is likely to want alternatives to, such as introducing uncomputable numbers.
Just change your conclusion! =P
LikeLike
I’m fine with students seeking to find a flaw in the real number system; they won’t find one, but they’ll learn a lot from trying!
John, you clearly don’t like the constructions of the real number system that you’ve seen (to the point where it appears that you think it’s the wrong number system). But I’m puzzled that you hate Dedekind cuts and like Conway’s surreal numbers, when Conway’s theory is Dedekind’s theory on steroids! My favorite way to construct the reals is via Cauchy sequences of rational numbers. You’d probably prefer the Faltin, Metropolis, Ross, and Rota method of constructing the real numbers. (There’s also some German mathematician who wrote an article about constructing the real numbers via decimal expansions, which you’d probably like even more, but I can’t locate the reference.)
I’m not convinced that any particular construction of the real number system is here to stay, and it’s conceivable that the real number system will in future centuries be supplanted in importance by something we can’t even imagine now. But it’s not going to be replaced by the literal decimal system. And that was the point I was trying to make.
LikeLike
In explaining that 0.999…….=1. Why not just explain that
1/3 = 0.333……
2/3 = 0.666……..
0.333…….+0.666……=0.999……..
but as 1/3 +2/3 = 1
then 0.9999……=1
LikeLike
I should’ve mentioned that as part of the background for Endnote #2. Some students who are shown that proof find it unsatisfying, but according to Joerg they find the explanation more convincing when it’s reinforced by more examples, involving not just 1/2 and 2/3 but 1/9, 2/9, etc. What has your experience been? (I don’t teach high school, so everything I say about pre-college pedagogy should be taken with a shakerful of salt!)
LikeLike
A response I have often heard from students who don’t agree that .999….= 1 is “you’ll never get there no matter how many 9’s you have.” Our intuition fails us when we deal with infinity, because we’ve never experienced infinity. It’s impossible to even visualize .999…. One theory that I have is that some people will visualize an animation of .999…. as a long string of 9’s with more 9’s being added on as time goes by. If they are using this type of animation to visualize .999…., then they are correct, we’ll never get to 1. I’m not sure if this comment is very useful in helping students’ understanding, but perhaps if we can try to understand why some can’t swallow our claim of .999… = 1, we can start towards a better understanding.
LikeLike
Thank you for your interest in explaining 0.999… to the world. I am one of the doubters/disbelievers. As I see it (and I certainly may be incorrect), there is a subtle difference between a) “the sum of (9/10)^n as n starts at 1 and goes on to infinity” and b) the symbol 0.999…
Following exactly the “command” of the sigma function symbol, a) tells me that I should add 9/10 + 9/100 + 9/1000 + an infinite number of consecutively smaller terms. Thus I will be carrying out a never-ending process. In my mind, a) does not represent a number on a number line; it is a process.
In my mind, b) is also a not number on a number line. It is a symbol that has been defined to represent the limit of a). But the limit of a) is 1. So why did mathematicians not simply stick to writing “1” (a number on a number line) as the limit of a)? I believe that one of the reasons is that it is a lot easier to write 0.999… than 9/10 + 9/100 + 9/1000 + … If decimal notation had not been introduced, then we would not have this issue. But we do have decimal notation, and just as it made for a convenient symbol for the limit of a) above, it also makes many calculations (such as adding fractions with different denominators) much easier. And that gets to the heart of the problem, I think. Decimal notation is a convenience, but it is never a necessity. But once that convenience was put in use, it created problems.
For instance, 21/9 is a number. I do not believe that it asks us to carry out a division; we use other symbols for that (none of which I have on my keyboard, but you know the ones: the dots above and below the horizontal line, and the long division symbol with the vertical line (or close parenthesis) followed by the horizontal line going off to the right from the top). If we stick with non-decimal notation and carry out long division on 21/9, we get 2 remainder 3, or 2 and 3/9, or 2 and 1/3. But once we decide that we must use decimal notation, we run into problems. Now we are forced to write 2.333333… There is no end to the threes because decimal notation cannot exactly describe the number 1/3. There is no need to have have decimal notation exactly describe 1/3, other than the fact that we like decimal notation, and because we like it, and want to use it, there must be a way to make it exactly describe 1/3. So we say that the symbol 0.333… will be defined as the limit of the sum of 3/10 + 3/100 + 3/1000 + …
Here we have three distinctly different terms all of which are subtly different
a) the number 1/3
b) the unending process of finding the sum of (3/10)^n as n starts at 1 and goes on to infinity
c) the symbol 0.333…
If there were no decimal notation, the symbol 0.333… would not exist, and we would say that the limit of b) is the number 1/3.
I put a lot down, but I would like to know your thoughts.
LikeLike
Thanks for writing, Tom! I think that you and I (that is, you and the mathematical mainstream) agree more than you realize; the main difference revolves around conventions for what symbols mean. Mathematicians insist that infinite decimals are all tacitly defined using the limit concept. In particular, .333… (your (c)) is by mathematicians’ definition equal to not the process (b) but rather the real number that the process (b) approaches in the limit, which is 1/3 (your (a)). (For an informal discussion of the limit concept, see my earlier essay on .999…) What’s lost when you define infinite decimals via the limit concept is the appealing concreteness of decimals-as-decimals; what’s gained is something that’s harder to describe. Anyone else want to jump in here and continue this answer to Tom’s question? I’d welcome fresh perspectives from teachers in the trenches who deal with this kind of dissidence much more often than I do.
LikeLike
Thank you for your quick response. My question to your response has to do with your use of the words “… the real number that the process b) approaches in the limit …”. In everyday life “approaches” means “gets near to”. So, taken at face value, I think that you are telling me that the process b) gets nearer and near to, but never reaches, the limit. Am I understanding you correctly? If so, then I agree with you that the limit is never reached, and that is why I do not accept that any repeating decimal every exactly equals the real number that is its limit. Defining 0.333… as 1/3 is an approximation, not an equality.
I believe that my stance is supported by the following philosophical conundrum:
Let x = 3/10 + 3/100 + 3/1000 +… + 3/(10^{n-1}) + 3/(10)^n
then 10x = 10(3/10) + 3/10 + 3/100 +…+ 3/(10^{n-1})
then 10x – x = 10(3/10) – 3/(10^n) = 10(3/10) – [(3/10) – (1/10^{n-1})]
or
x = {10(3/10) – [(3/10) – (1/10^{n-1})]}/(10 -1) EQ. A
If you re-write the standard formula for calculating the sum of an finite geometric series
[s_1 – {(s_1)x(r^n)}}/(1 -r),
you will get
Sum = {10(s_1) – [(s_1)x(1/r^{n-1})]}/(10 -1) EQ. B
If r = 1/10 and s_1 = 3/10, then equations A and B are identical.
The interesting part comes when you look at the subtracted term (3/10)x(1/10^{n-1}) . That is always equal to the last term of the finite series. (So that the sum of 3/10 + 3/100 + 3/1000 = [10(3/10) – (3/10)x(1/10^2)]/9 = [3-(3/1000)]/9 = 333/1000.)
But that subtracted term (always the last term in the series) is also the term that must be equal to 0 for the equation for the sum of a finite geometric series to collapse into the equation for the sum of an infinite geometric series:
Sum = s_1/(1-r)
and if r = 1/10, then
Sum = s_1/( 1 – 1/10) = 10(s_1)/(10-1) EQ. C (if s_1 = 3/10, then {10(3/10)}/9 = 1/3)
But how can that be? How can there be a last term in an infinite series? And if there is a last term, how can it have the value of 0?
I submit that you can’t have there be an infinite series and have a last term. I also submit that you can’t write off that last term by saying it approaches 0, because a single term can’t approach zero; it either is 0 or is not zero.
In other words, to make both the equation for the sum of an infinite geometric series and the standard let x = 0.333…, then 10 x = 3.333… etc. “proof” work, you have to make an approximation, or you have to accept a philosophical dilemma – the last term of an infinite series (that does not exist) is equal to 0 (which it cannot possibly be).
Again, thanks for listening and pondering my questions.
LikeLike
Sorry for the lag in my reply. I agree with almost everything you say — almost to the point where my main disagreement with you is the fact that you call yourself one of the doubters/disbelievers! If you accept that the numbers 3/10, 33/100, 333/1000, etc. converge to the number 1/3 (that is, if you believe that for any two-sided neighborhood of the number 1/3, all but finitely many of the terms of the sequence lie inside the neighborhood), then you’ve aligned yourself with the mainstream, in all matters save terminology and notation. If you believe that the sequence 3/10, 33/100, 333/1000,… converges to 1/3, then you believe that the sum of the infinite series 3/10 + 3/100 + 3/1000 + … equals 1/3, in the sense that mathematicians mean that statement (because by definition, the value assigned to an infinite sum is the limit of the sequence of partial sums). When we say “3/10 + 3/100 + 3/1000 + … = 1/3”, we don’t mean that you ever reach the limit; just that you get closer and closer.
(And if you object “But then they’re never equal!”, the response is “The definition of 3/10 + 3/100 + 3/1000 + … isn’t any particular term of the sequence of partial sums, or even the sequence as a whole, or the process that gives rise to it; it’s the LIMIT of that sequence.” And if you reply “But then why don’t you use the word LIMIT anywhere in the formula?!”, they reply “That’s what +… means to us.”)
This still leaves us with the question, why does 0.333… mean 3/10 + 3/100 + 3/1000 + … (or, if you prefer, 0 + 3/10 + 3/100 + 3/1000 + …)? The answer is, mathematicians adopted this as a convention, centuries after the decimal system was introduced. The point I was trying to make in my article is that you can do a style of mathematics in which this convention is absent. It’s self-consistent, and some people will find it intuitively satisfying (because in this system .999.. and 1.000… aren’t equal). But it’s neither as pretty nor as useful as the real number system, so I tend to view it as a pedagogical way-station on the road to understanding the real number system.
LikeLike
Great topic!
As Amy points out, the 1/3, 2/3, 3/3 argument, and/or the similar one for 1/9, 2/9, … are indeed convincing to many. But it is interesting that those who are not convinced don’t usually object to 1/3 = 0/333… It is worth pointing out to them that their views are inconsistent, and that mathematicians aim for consistency.
I agree with the principle behind your idea of “good humored reticence”, as it is a waste of everyone’s time to try to answer questions that students don’t have, or can’t have. However I don’t think it applies here, because just about everyone is interested in this particular question. I think nothing is lost by saying “mathematicians believe that .999… = 1, but many people don’t agree.” This is straightforward, intellectually honest, and opens the door to further discussion, including the idea that “you never get there” and encapsulation.
I do think that the distinction between “numeral” and “number” is pedantic, and uninteresting to non-mathematicians. If you insist on having that conversation, maybe start by discussing “this is not a pipe”, which at least is fun.
In a formal mathematical presentation, definitions come first. But pedagogically, it’s exactly the wrong approach. In learning new ideas, definitions had better come after the student has some understanding of the subject under discussion. Concerns about terminology are valid, but only after there’s some understanding, not as an alternative or predecessor to understanding. Solving a teaching problem with a terminology solution is almost always an admission of defeat.
A more realistic strategy to lay the groundwork for a conversation about 0.999… is to talk about numbers having different representations, e.g. 0.25, 0.2+0.05, 1/4, 3/12, and (.5)^2 all represent the same number. That at least is more immediately useful in pre-college education than picky points about language.
Finally, I should mention that in most schools, the sum of a convergent geometric series is among the first exposures students have to the concept of limit. This is typically seen in 10th or 11th grade. Including the question about 0.999… in that unit helps spice things up, and is a good way to make the discussion of the meaning of an infinite sum interesting to pretty much all students.
LikeLike
Pingback: Carnival of Mathematics 145 | The Aperiodical
One comment that nobody made is that I misinterpreted that data I got from my students! In my opening I wrote “The students agreed that .999… is the limit of the sequence .9, .99, .999, etc., and they also agreed that the limit of that sequence is 1.” But if you read endnote #1 carefully (as evidently I failed to do!), you’ll see that most of the class disagreed with the first of the two assertions! That is, they refused to accept the convention (universally subscribed to by mathematicians) that .999… MEANS the limit of the sequence. And the reason they didn’t accept that convention (I think!) is that to them, it isn’t a convention. If .999… already means something to you, you’re not going to let some bunch of mathematicians tell you that it means something else.
I’d love to hear more from actual pre-college teachers (and recent “converts”) about this. But here’s a metaphor that occurs to me: if you want someone to change out of ill-fitting clothes, you’ve got to make new clothes available AND you’ve got to help them to realize that the old clothes are uncomfortable. The latter requires a certain amount of discretion, perhaps even indirection. Don’t say “That shirt is too small”; have the kid play some sports, and let her see for herself that the too-tight shirt is inhibiting her movement. In mathematical terms, the analogous course would be posing challenges like “What’s the decimal expansion of 1.000… minus 0.999…?” that expose the inherent tensions of the literal decimal system. The trick is doing this in a gentle way that doesn’t appear to be bullying the student into accepting the equality of the two numbers before she’s ready.
LikeLike
Pingback: Band saw blades, bedbug zappers, rubber bands and me |
Pingback: Denominators and Doppelgangers |